Extensions of the Zwart-Powell Box Spline for Volumetric Data Reconstruction on the Cartesian Lattice
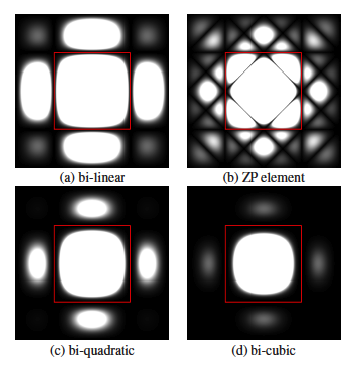
In this article we propose a box spline and its variants for reconstructing volumetric data sampled on the Cartesian lattice. In particular we present a tri-variate box spline reconstruction kernel that is superior to tensor product reconstruction schemes in terms of recovering the proper Cartesian spectrum of the underlying function. This box spline produces a C2 reconstruction that can be considered as a three dimensional extension of the well known Zwart-Powell element in 2D. While its smoothness and approximation power are equivalent to those of the tri-cubic B-spline, we illustrate the superiority of this reconstruction on functions sampled on the Cartesian lattice and contrast it to tensor product B-splines. Our construction is validated through a Fourier domain analysis of the reconstruction behavior of this box spline. Moreover, we present a stable method for evaluation of this box spline by means of a decomposition. Through a convolution, this decomposition reduces the problem to evaluation of a four directional box spline that we previously published in its explicit closed form [8].
 Top
Top
- Entezari, Alireza
- Möller, Torsten
 Top
Top
Category |
Paper in Conference Proceedings or in Workshop Proceedings (Full Paper in Proceedings) |
Event Title |
IEEE Visualization (VIS) 2006 |
Divisions |
Visualization and Data Analysis |
Subjects |
Computergraphik |
Event Location |
Baltimore, Maryland |
Event Type |
Conference |
Event Dates |
Oct 29 - Nov 3 |
Date |
October 2006 |
Export |
 Top
Top



