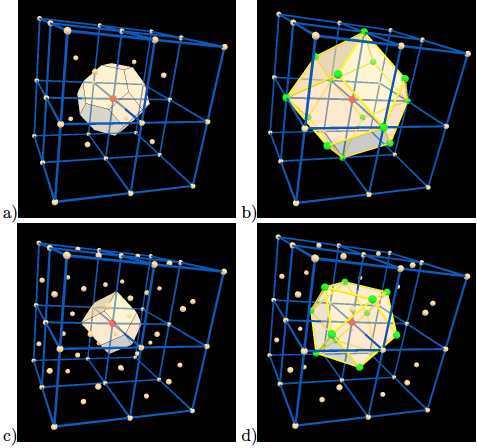
In this paper we introduce reconstruction kernels for the 3D optimal sampling lattice and demonstrate a practical realisation of a few. First, we review fundamentals of multidimensional sampling theory. We derive the optimal regular sampling lattice in 3D, namely the Body Centered Cubic (BCC) lattice, based on a spectral sphere packing argument. With the introduction of this sampling lattice, we review some of its geometric properties and its dual lattice. We introduce the ideal reconstruction kernel in the space of bandlimited functions on this lattice. Furthermore, we introduce a family of box splines for reconstruction on this sampling lattice.We conclude the paper with some images and results of sampling on the BCC lattice and contrast it with equivalent samplings on the traditionally used Cartesian lattice. Our experimental results con�rm the theory that BCC sampling yields a more accurate discrete representation of a signal comparing to the commonly used Cartesian sampling.

Top